三角比の相互関係・鈍角の場合
鈍角の三角比の相互関係
前回から、三角比を \(90°\) を超えても定義しました。
単位円による定義ですね。
角の範囲が \(90°\) を超えても、三角比の相互関係の公式が成り立ちます。
\(0° \leqq \theta \leqq 180°\) で
- \(\sin^2 \theta +\cos^2 \theta =1\)
- \(\tan \theta=\displaystyle \frac{\sin \theta}{\cos \theta}\)
- \(\tan^2 \theta+1=\displaystyle \frac{1}{\cos^2 \theta}\)
※実は、 \(0° \leqq \theta \leqq 360°\) で三角比の相互関係は成り立ちます。
証明は省略します。
\(90°\) を超えた三角比を、そもそもどのように定義したのか、
ということをもとに、三平方の定理レベルで \(90°\) 未満のときとほぼ同様に示すことができます。
例題1
\(0° \leqq \theta \leqq 180°\) で \(\tan \theta =-2\) のとき、
\(\sin \theta\) と \(\cos \theta\) の値を求めなさい。
解説
三角比の相互関係を用いて、ガツガツ計算することもできますが、
やはり図示することが大事です。
\(\tan \theta=\displaystyle \frac{y座標}{x座標}\)
なので、これは \(1\) 次関数の傾きが与えられたのと同じことです。
原点を通る、傾き \(-2\) の直線 \(OP\) をかきましょう。
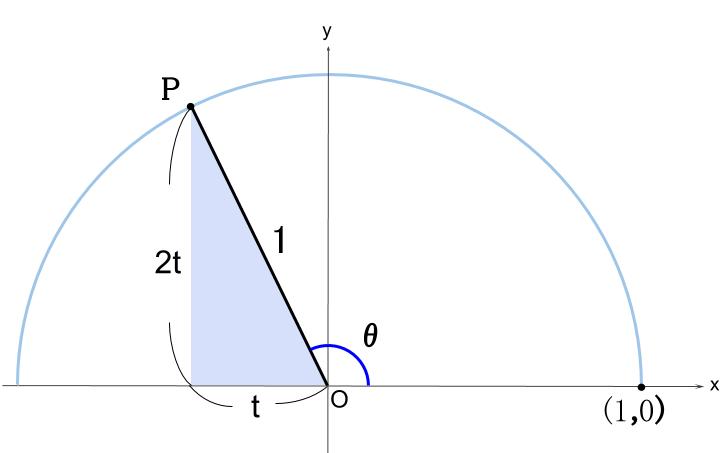
水色の直角三角形で三平方の定理を用います。
\(1^2=t^2+(2t)^2\)
\(t \gt 0\) より、\(t=\displaystyle \frac{1}{\sqrt{5}}\)
点 \(P\) の座標から、\(\sin \theta\) と \(\cos \theta\) が決まります。
\(\sin \theta=\displaystyle \frac{2}{\sqrt{5}}\)
\(\cos \theta=-\displaystyle \frac{1}{\sqrt{5}}\)
※\(\cos \theta\) は、\(-t\) です。符号に注意しましょう。
※単位円でない図示をしても解けますが、単位円をおすすめします。
例題2
\(0° \leqq \theta \leqq 180°\) で \(\sin \theta=\displaystyle \frac{3}{5}\) のとき、
\(\cos \theta\) と \(\tan \theta\) の値を求めなさい。
解説
図示です。
単位円がおすすめです。
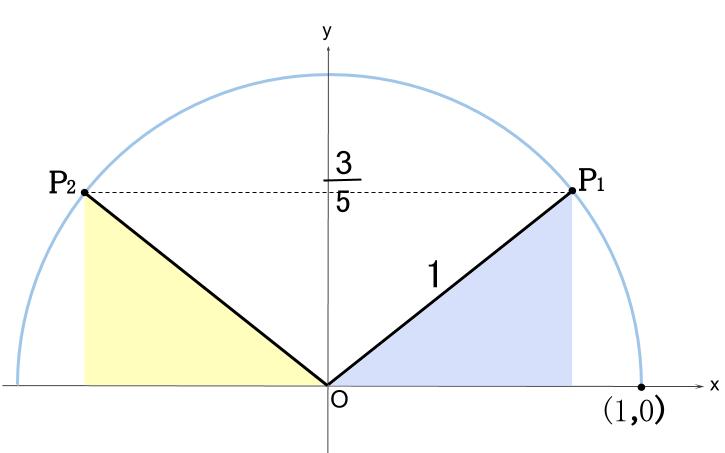
\(\sin \theta\) の値は \(y\) 座標です。
つまり、\(\sin \theta=\displaystyle \frac{3}{5}\) となる点 \(P\) は \(2\) つあります。
\(P_{1}\) の \(x\) 座標は、水色の直角三角形で三平方の定理を用いて、\(\displaystyle \frac{4}{5}\) です。
※ \(3:4:5\) の直角三角形であることを用いても可
よって、
\(\cos \theta=\displaystyle \frac{4}{5}\)
\(\tan \theta=\displaystyle \frac{y座標}{x座標}\)より、
\(\tan \theta=\displaystyle \frac{3}{4}\)
また \(P_{2}\) の \(x\) 座標は、クリーム色の直角三角形で三平方の定理を用いて、\(-\displaystyle \frac{4}{5}\) です。
\(\cos \theta=-\displaystyle \frac{4}{5}\)
\(\tan \theta=-\displaystyle \frac{3}{4}\)
よって、以下のように \(2\) 通りの答えを解答とします。
\(\cos \theta=\displaystyle \frac{4}{5}\)、 \(\tan \theta=\displaystyle \frac{3}{4}\)
または
\(\cos \theta=-\displaystyle \frac{4}{5}\)、\(\tan \theta=-\displaystyle \frac{3}{4}\)